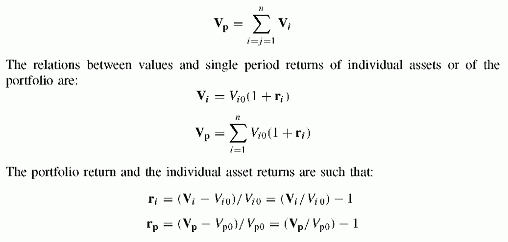Business — Banking — Management — Marketing & Sales

This appendix details the usual demonstration of decreasing portfolio return volatility when the number of assets gets large. The portfolio return volatility decreases to a floor set by general risk. When the asset returns are independent, the portfolio return volatility decreases to zero. When there is a common factor affecting all individual returns, the floor is the general risk depending on the correlation level between individual asset returns. By contrast, the portfolio value volatility increases with the number of assets. The sensitivity of the value volatility to the number of assets increases with the average correlation between asset returns.

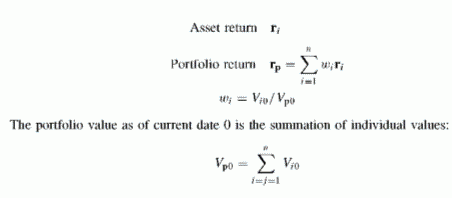
Asset and Portfolio Returns

The portfolio return is the weighted summation of individual returns, the weights being the ratios of the individual asset values to the initial portfolio value:

The first index relates to the individual asset, or to the entire portfolio, and the second index designates the date 0 as the current date. The portfolio value at a future date is random and is the summation of individual values:

Using the standard notations, we can show that the portfolio return variance converges rapidly towards a floor when the number of assets gets large enough. The floor is zero when returns are independent and is proportional to the average portfolio covariance when the correlation is positive.

Portfolio Return Variance

The first term gets close to zero when N grows, while the second term results from the correlation only, and remains constant. Consequently, the portfolio return variance decreases rapidly to this floor when the number of assets grows. The variance of the return collapses: a²(r_p) = p x a² when N becomes large, which is the systematic undi-versiiable risk of the portfolio return due to the volatility of the common index driving all individual asset returns. The same property holds when covariances and variances are unequal, but is more dificult to demonstrate.